Using a Siemens star ist a great way to measure the MTF of a camera system. We use this method for years and see that it is much more robust against image enhancement and other influences than a slanted edge.
A question we get from time to time is: “What size of chart do I need to measure my camera with X pixel?” or “I would like to have this chart, what is the maximum pixel count my camera can have?” In this TechNote, I would like to explain shortly how this is calculated.
Nyquist Frequency
As a first step, we calculate the smallest radius we would like to use.
(Details of the method can be found here »)
1. The spatial frequency, expressed in cycles per pixel is calculated according to this equations:
![]()
![]()
2. Expressed in cycles der pixel, Nyquist frequency equals 0.5.

3. Combining these equations and solving for the radius, we get:
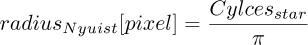
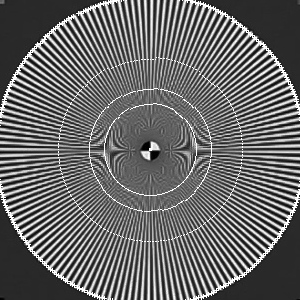
So you see that the radius of Nyquist frequency only depends on the star itself, not on the pixel count of the camera. This can also be seen easily. These are two images, taken by the same camera, both analyzed with the iQ-Analyzer, our software solution for image quality evaluation. Both images are taken with the same camera. One in Photo-Mode (14 Megapixel), the other in video mode (frame extracted in the video module of the iQ-Analyzer, both analyzed in the resolution module). You see that the radius of Nyquist frequency (the inner circle) is equal for both modes, but you see more details of the Siemens star.
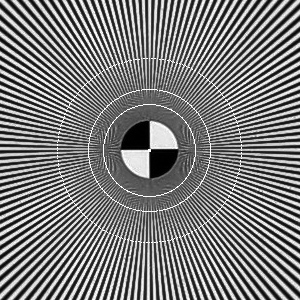
So with this in mind, we see that the limit is the size of the center marker in the Siemens star (so the area that can be resolved in the production process) relative to the image height.
So how to calculate the limit now?
We know that the maximum allowed diameter of the center marker in the image is ~92pixel (with a Siemens star of 144 periods). So what we need to calculate is the relative size of the star in the image.
Example: TE253 9x in A1066
In A1066, the complete chart is 810 mm in height. We have three Siemens stars in height, so each star has 270 mm in height. The (current) limit in producing the siemens star allows a diameter of the center marker of 12 mm.
So the limit is, that 92 pixel make 12 mm / 810 mm of the chart. With the assumption, that the 810 mm fill exactly the image, we know that the maximum height of the image in pixel is 6210 pixel. With an aspect ratio of 3:2, we get a maximum pixel count of 57.8 megapixel.
This is quite a lot, but we have to consider some more things:
For this calculation, we assumed that we only want to measure up to exactly Nyquist frequency. Normally, we also want to have a look at higher frequencies (even if this is mostly aliasing region). So measuring up 25% over Nyquist, reduces the maximum pixel count to ~37 megapixel. Another point: A lot of cameras have a 4:3 aspect ratio. In this case, we have again a slightly lower maximum pixel count.
Giving some additional headroom, we can clearly say, that a TE253 9x with 144 cylces can be used to measure cameras with up to 30 megapixel.
Want it another way around?
We can also inverse the calculation. What is the minimum size we need to analyze an HD- camera?
With the given 1080 px in height, we know that the center marker can be 92 px / 1080 px of the image. So the marker can be ~8.5% of the image. If this equals 12 mm in the chart, we have a chart size of ~141mm. Again, this is the absolute theoretical maximum, in real world we prefer to use a larger chart.
ua